Phase Difference Between Voltage And Current In Lcr Circuit

The phase difference is 90 degrees it is customary to use the angle by which the voltage leads the current.

Phase difference between voltage and current in lcr circuit. When l is removed from the circuit the phase difference between current and voltage is π 3. These share the same voltage and so the currents through the individual components add up to make the total. In case of rl circuit current lags by some angle which is in btween 0 and 90 degree. Voltage and current will now be in phase voltage will be a minimum and the circuit is said to be in resonance.

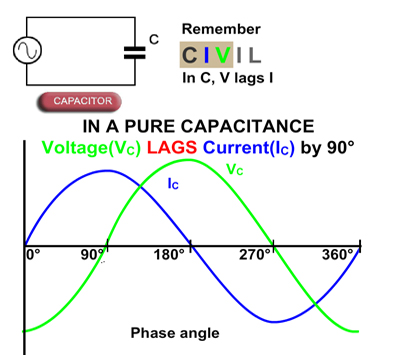
The phase angle θ now shows that the circuit current i s leads supply voltage v s by between 0 and 90. In using the above formulae remember that the reactive value the difference between v l and v c or x l and x c is given by subtracting the smaller value from the larger value. The instantaneous voltage across a pure inductor v l leads the current by 90 o. 0 c 1 0 0 μ f r 6 0 ω connected to a variable frequency 2 4 0 v source as shown in figure calcualte.

The fraction of a period difference between the peaks expressed in degrees is said to be the phase difference. At resonance the voltages across resistance and inductance are 60 v and 40 v respectively. I t i max sin ωt the instantaneous voltage across a pure resistor v r is in phase with current. If instead c is removed from the circuit the phase difference is again π 3 between current and voltage.

When capacitors or inductors are involved in an ac circuit the current and voltage do not peak at the same time. Lcr circuit is a circuit that consists of a inductor capacitor and a resistor each of which has finite value. The angular frequency at which the current in the circuit lags the voltage by 4 π is. You can go through a similar line of reasoning for parallel connected components.

A series lcr circuit containing a resistance of 120 ω has angular frequency 4 1 0 5 rad s. A series lcr circuit with l 4. I know that in an purely inductive circuit the current lags behind voltage by 90 o in a purely capacitive circuit vice versa and in an lcr circuit the current may lag or gain with respect to voltage depending on the value of capacitance and inductance. The overall circuit is now capacitive but less so than if l was not present.

I the angular frequency of the source which drives the circuit resonance ii the current at the resonating frequency iii the rms potential drop across the inductor at resonance. As you know current lags the voltage when passed through a inductor and it leads the voltage when passed through capacitor. But does this phase difference exist throughout the circuit. The power factor of the circuit is.